Breaking Down the Arc Length Formula

The arc length formula is Arc Length = Radian Angle x Arc Radius or Arc Length = (Degree Angle/360) x 2π x Arc Radius, depending on whether you're measuring in radians or degrees.

That said, if you don't already have the arc radius or know the role of pi in this situation, you'll want to keep scrolling for more details.

Before we can calculate arc length, we must first understand how to calculate circumference, as well as the relationship between circumference and a circle's (or arc's) radius. This relationship is said to have been discovered by the ancient Greeks more than 2,000 years ago, who assigned the Greek letter π (pi) to describe it.

If we have a circle on a piece of paper and draw a line segment straight across through its center and to the other side, we create what is known as a diameter. The straight line distance directly to the center is called the radius, which is always exactly half the length of the diameter.

Finding π

The Greeks also found that, by creating a diameter line segment out of string and wrapping it around the curved line edge of the circle, the circumference (or length around the circle) was equal to slightly more than three times the chord length of their string.

They gave this value the name π (Pi) and calculated more specific values for this ratio.

The true value of π uses and infinite, non-repeating number of decimal digits, but for our purposes we can say that π is equal to 3.14159. The exact value of the number is not all that important, since most calculators include a π key with a more precise figure.

With all that in mind, the formula for calculating any circle's circumference is the length of the diameter chord length times π (Dπ). You may also see this expressed as two times the radius times π (2rπ), but the result will be the exactly the same using either version of the formula.

For example, if a circle has a diameter of 4 inches, the circumference will be:

or, since we know the radius is half the diameter (4 in / 2 = 2 in):

As stated, an arc is a fraction of a circle, and an arc will have the same radius length of its corresponding. These two facts are the most important to keep in mind when we try to calculate the arc length.

As long as the curve of an arc is known, and the location of the center point is know, we are able to find the arc length, although additional tools like a ruler and protractor may be required.

Measure the Radius

If we know the center point of our arc, then we can find the length of the radius by drawing two line segments from the edge of our arc and into the center point. Use a ruler to measure the straight line distance between the the arc to its center, and that's our radius.

We can use our circumference conversion formula above to see what the circumference length would be if our arc was a full circle, but first, we will have to figure out what the fractional value of the arc is. To do that, we need an angle value.

The central angle is the angle formed by drawing the radii line segments we created in the last step, and this angle measurement is the final value needed to find arc length.

If your textbook or instructor did not provide a central angle, you will have to draw two radii at the edges of the arc and then use a protractor to measure the angle where they meet at the center point.

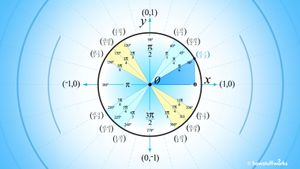
You can take this measurement in either degrees or radians. If the central angle is greater than 180 degrees (π radians), you may want to measure the opposite side of the angle and then express it as your measurement plus 180 degrees.

Degrees vs. Radians

While degrees and radians are units used to measure the same thing, they serve slightly different purposes. One degree represents 1/360 of a circle, while a radian is a function of the ratio between radius and circumference. For this reason, a radian is equal to 1/2π.

A full circle is always 2π radians because, as you may recall, circumference is equal to 2π times the radius of the circle. We also have two handy formulas that can be used if you ever need to convert degrees to radians, or vice versa.

Fractions of a Circle

Now that we know that central angle can be used to express a fraction of a circle, and that radius has a close relationship with arc length, we have just about everything we need to calculate the arc length.

To better visualize fractions of a circle, think of a 90 degree (1/2 π radians) angle as a quarter circle, 180 degrees (π radians) as a half circle, and 360 degrees (2 π radians) as a full circles. Of course, real world examples will often be fractional values in between these neat angles.

Remembering the Arc Length Formula

Once you've found the central angle, you can plug it into the circumference formula from earlier to create and arc length formula. To obtain the arc length formula for degrees, take your central angle value, divide it by 360 and then multiply the result by our circumference formula.

If you calculate the arc length using radians, the process is a bit simpler. Since a radian value is a fraction of 2π, and we need to multiply by 2π in order to calculate the arc length, the two cancel out. We are left with this formula:

Now we can use all of this information to run through an actual problem. Let's take our circle from the previous example with a 4-inch diameter (2-inch radius) convert it into a fractional arc. We have measured the central angle or the arc and found it to be 136 degrees (2.374 radians).

Finally, this is all the information we need in order to calculate the arc length!

First, let's use the arc length formula for degrees: (Degree Angle/360) x 2π x Arc Radius

Now, we can run the same problem again using radians for our central angle.

As you can see, determining arc length is easy as long as the radius and central angle are known. Our arc length formula can be reliably used to convert both degrees and radians, and we get two arc lengths with the same value.