Finding Factors of 36: Factor Trees and Prime Factorization

In mathematics, a factor is a number that can be divided into another number without a remainder. You'll most often encounter factors in the following way: You'll receive a number (from your teacher, a homework problem, a test or a situation), and you will be tasked with finding the factors of that number.

From lowest to highest, the factors of 36 are 1, 2, 3, 4, 6, 9, 12, 18 and 36. These are all the numbers you can divide 36 by and have whole numbers with no remainders.

That's it, those are all the factors of 36! There aren't any others, which makes this a good example to illustrate the concept of factors. However, there are lots of different ways to use factors, or to categorize them.

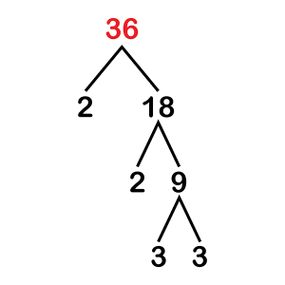
Here are some examples that show how all the factors of 36 work:

36 ÷ 1 = 36

In this case, we're working with both the highest and the lowest value factors of 36. So, when we divide 36 by 1, we get 36. This leaves no remainder, and the number we started with: a good way to begin.

36 ÷ 2 = 18

36 divided by 2 is 18. Again, there's no remainder, as 18 is half the value of 36. Conversely, 18 multiplied by 2 is 36.

36 ÷ 3 = 12

36 divided by 3 is 12. Running the equation the other way, we can see that 12 times 3 is 36. As usual, there are no remainders, and thus 3 is the third factor of 36.

36 ÷ 4 = 9

36 divided by 4 is 9. And so of course, 9 times 4 is 36.

36 ÷ 6 = 6

6 times 6 is 36; 36 divided by 6 is (you guessed it!) 6. As always, there are no remainders.

36 ÷ 9 = 4

This might give you a bit of deja vu, and if so, good! As you'll recall from a few equations ago, 4 times 9 is 36. Thus, 36 divided by 4 is 9.

36 ÷ 12 = 3

Once again, we're covering familiar ground. 12 times 3 is 36; 36 divided by 12 is 3.

36 ÷ 18 = 2

If this is seeming repetitive, that's okay. Sometimes math is about repetition, which helps with memorization, and can also help the concept really sink in. We want to be thorough, so we're noting that 18 goes into 36 twice.

36 ÷ 36 = 1

Now we're right back where we began! Any number divided by itself is 1, and so we're including 36 as one of the factors of 36.

Now that you know the factors of 36 — backwards and forwards — you might feel like you've got the concept covered. But what if you didn't know the factors of 36 to begin with? In order to find factors from scratch, you need to do a little division.

In order to determine the factors of 36, you need to divide the number (36) by all the natural numbers from 1 to 9. Some of them, like 1, 2, 3, 4, will divide evenly into 36. But notice what happens when you hit 5: There is a remainder.

5 x 7 = 35. The closest you can get to 36 using 5 is 7, and then you get a remainder of 1. Thus, 36 ÷ 5 = 7 with a remainder of 1.

Since you can't divide 5 evenly into 36, 5 isn't a factor. Note that the same is true of 7 and 8. Now you've determined all the factors of 36 using some basic division.

Another way to list factors is by writing them as pairs. These factor pairs are numbers that, when multiplied together, give you 36.

Positive Factor Pairs

Since we've already determined all the factors of 36, we can now pair factors according to their place in an equation that results in 36.

Note that these factor pairs of 36 can also be listed in reverse, e.g. 4 x 9 = 9 x 4.

Negative Factor Pairs

So far, we've been talking about positive factors of 36 only. But if you recall, when you multiply two negative numbers together, you get a positive number. That means that you can also determine a list of negative factor pairs as well.

Thus, the negative factors of 36 essentially mirror the positive factor pairs of 36. For all the positive factors, you can make them negative, and you will have your answer.

The prime factors of 36 are 2 and 3. That's it.

But we should clarify our terms, since prime factorization isn't very useful unless you know what prime numbers are.

A prime number is a natural number that is greater than 1, and which cannot be divided evenly by another number other than itself or 1. Thus, 2, 3, 5, 7 and 11 — to name a few — are all prime numbers.

Note that the other numbers that divide 36 — the factors of 36 that aren't prime — are called composite numbers. A composite number is a number that has more than two factors.

Since we've already established the factors of 36, we simply need to see which factors of 36 are also prime numbers. In this case, of the factors already listed, we can see that 2 and 3 are the only prime factors.

Besides the method of comparison we just mentioned, there is a specific process by which prime factorization can be solved.

You can write the prime factorization of 36 as:

You could also write it as:

In both cases, you have established the prime factors of 36 as 2 and 3.

You can also determine the prime factors of 36 using a factor tree. Factor trees are a way of visualizing the prime factorization method listed above.

To draw a factor tree, start with your given number, which is 36. Then, follow the prime factorization steps outlined in the previous section. The procedure is the same, except you write each of the factor pairs underneath the previous numbers.

Take 36 ÷ 2 = 18: This means you write 2 and 18 under 36. Under 18, you write 9 and 2. Under 9, you write 3 and 3. And now you have a visual shorthand that shows you the prime factors of 36.

You could also divide 36 by different factors, such as 4 and 9, and then divide each of those until you reach all the prime factors. In this case, you would still end up with the same prime factors of 36.

Another type of math problem you will likely encounter is to determine the common factors of two numbers. In order to answer this question, we can refer back to the methods we've already learned.

For example, what are the common factors of 36 and 14?

We already know that the factors of 36 are 1, 2, 3, 4, 6, 9, 12, 18 and 36. Using the methodology outlined above, we can see that the factors of 14 are 1, 2, 7, and 14.

Therefore, the common factors are 1 and 2. 36 and 14 have only two factors in common.