Integers, Integer Properties and the Role of Zero

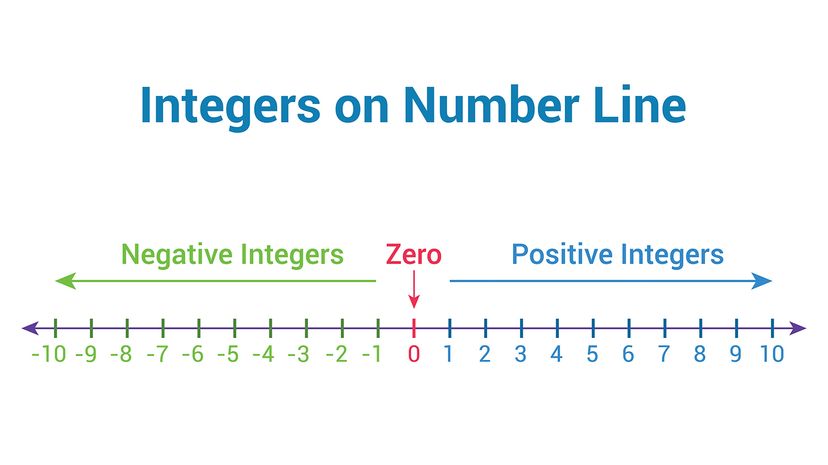
Positive and negative whole numbers on a number line — You can represent integers on a number line to easily see each one's distance from zero. Dream01 / Shutterstock

Integers are natural numbers (or whole numbers) that stem from the Latin word meaning "intact." In other words, any two integers will be rational numbers. A rational number is a value without fractional part or decimal remainders.

These counting numbers are some of the most common values for both complex arithmetic operations and simple real-life applications using addition and multiplication.

Contents

Positive and Negative Numbers
How Are Integers Shown on a Number Line?
Is Zero a Positive Integer?
7 Properties of Integers With Examples

Positive and Negative Numbers

There are two basic types of integers: positive and negative integers. These include any natural number, including zero.

How Are Integers Shown on a Number Line?

Consecutive integers are visually represented on a number line with zero in the middle. Any negative integer value is shown to the left of zero, and positive integers lie on the right.

Positive Integers

On an integer number line, positive integers lie to the right of zero. Each tick mark to the right represents an increase of positive numbers by an absolute value of 1.

Negative Integers

Negative integers lie on the left side of zero. Although these represent negative value increments of negative numbers, the absolute values represent an equal distance from zero as their inverse positive integers.

Is Zero a Positive Integer?

Zero is grouped in with other whole numbers but is not considered a member of either positive or negative numbers. Zero provides the anchor on which both positive or negative integers on a number line are based.

7 Properties of Integers With Examples

The primary properties of integers include the following:

1. Closure Property

The set of integers is closed under addition and multiplication, meaning that the sum or product of any two integers is also an integer.

2. Associative Property

In integer addition and multiplication, the way in which numbers are grouped does not change the result. Here are two examples.

(a + b) + c = a + (b + c)

and

(a x b) x c = a x (b x c)

3. Commutative Property

The order of integers in addition and multiplication does not affect the result. Here are two examples.

a + b = b + a

and

a x b = b x a

4. Distributive Property

Multiplication distributes over addition for integers, meaning:

a x (b + c) = (a x b) + (a x c)

5. Additive Inverse Property

Every integer a has an additive inverse –a such that:

a + (–a) = 0

6. Multiplicative Inverse Property

Every nonzero integer a has a multiplicative inverse 1/a, but since 1/a is typically not an integer, this property mostly applies to rational numbers.

7. Identity Property

The identity element for addition is 0, since a + 0 = a. The identity element for multiplication is 1, since a x 1 = a.

We created this article in conjunction with AI technology, then made sure it was fact-checked and edited by a HowStuffWorks editor.