Understanding Point Slope Form and the Applications in Algebra

Woman sitting in front of blackboard with chalk thought bubble — A linear equation can be expressed in three different ways: standard form, point slope form and slope intercept form. Today, we're asking you to make room in your brain for the second one as we dive into the point slope form formula. Justin Lewis / Getty Images

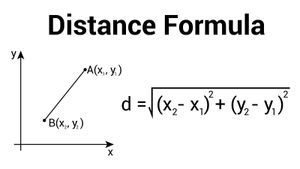
Algebra is the branch of mathematics that focuses on formulas, and one of its key concepts is the representation of linear equations, which describe straight lines.

Among the various forms you can use to express these equations, the point slope form is particularly useful if you want to understand the relationship between the slope of a line and the coordinates of points it passes through.

Contents

What Is Point Slope Form?
How to Use the Point Slope Form
Converting Point Slope to Slope Intercept Form
The Versatility of Point Slope Form
Relationship Between Point Slope Form and a Straight Line
Point Slope Form Calculator

What Is Point Slope Form?

The point slope form equation is a way to write the equation of a line when you know the slope of the line and the coordinates of one point on the line.

You express a point slope form equation as y – y ₁ = m (x – x ₁ ), where m represents the slope of the line, and (x₁, y₁) are the coordinates of the given point through which the line passes.

The point slope form is particularly useful when you have these two pieces of information and need to find the equation of the line.

How to Use the Point Slope Form

To illustrate how the point slope form works, imagine that you have a point on the line, say (3, -2), and the slope of the line, m = 4. Using the point slope formula, you can write the equation of the line as:

y + 2 = 4 (x – 3)

You can then simplify this equation to other forms, such as the slope intercept form, where the equation of the line is expressed as y = mx + b, with b being the y intercept, or the point where the line crosses the y axis.

Converting Point Slope to Slope Intercept Form

To convert the above point slope equation to the slope intercept form, you would simplify the equation to solve for y. Continuing from the previous example, that would look like this:

y + 2 = 4x – 12

y = 4x – 14

Here the slope (m) is 4, and the y intercept (b) is -14.

The Versatility of Point Slope Form

Deriving equations when you know one point and the slope aren't the only situations in which you can use point slope form. It can also be instrumental when you're trying to solve problems involving two points and you need to simplify a line equation.

If you're given two points — for example, (3, -2) and (1, 6) — you can first use the slope formula to find the slope:

m = (y₂ – y₁) / (x₂ – x₁)

In this case:

m = (6 – (-2)) / (1 - 3)

m = 8 / -2

m = -4

Now, using one of the points as the known point in the point slope formula, you can form and simplify the line equation.

Relationship Between Point Slope Form and a Straight Line

The point slope form of an equation is directly linked to straight lines in geometry and algebra. Mathematicians use this form specifically to define the equation of a straight line when they know the slope of the line and at least one point on the line.

This formulation is extremely helpful in algebra and calculus because it provides a straightforward method to write the equation of a line when solving problems that involve the relationship from one straight line to another straight line (also known as linear relationships).

Point slope form also serves as a foundational concept for more advanced studies involving linear equations and functions.

Point Slope Form Calculator

(X₁ Y₁) =
(X₂ Y₂) =

Slope m =

We create this article created in conjunction with AI technology, then made sure it was fact-checked and edited by a HowStuffWorks editor.