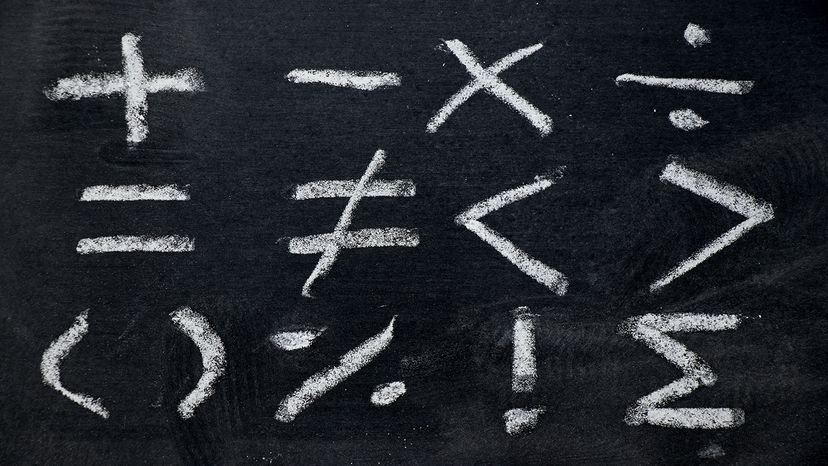
Key Takeaways
- The 11 basic symbols essential for writing mathematical equations are the plus (+), minus (-), equals (=), does not equal (≠), multiplication (×), division (÷), greater than (>), less than (<), greater than or equal to (≥), less than or equal to (≤), fraction (/), decimal (.) and percent (%) symbols.
- Each symbol has a specific function, forming the language of mathematics.
- Understanding these symbols is crucial for solving math problems.
Common math symbols give us a language for understanding, well, everything from budgeting to the nature of reality itself. Its building blocks are relatively simple. Even the most sophisticated mathematical equations rely on a handful of fundamental common math symbols.
Before you can solve the mystery of the Collatz Conjecture, figure out a square root or understand more complex algebraic symbols, you'll need to master the basic mathematical symbols that are necessary for writing a mathematical equation.
Advertisement
Advertisement

