How Special Relativity Works

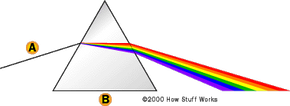
If you want to describe the universe as we know it in its most basic terms, you could say that it consists of a handful of properties. We are all familiar with these properties - so familiar, in fact, that we take them completely for granted. However, under special relativity many of these properties behave in very unexpected ways! Let's review the fundamental properties of the universe so that we are clear about them.

Space

Space is the three dimensional representation of everything we observe and everything that occurs. Space allows objects to have lengths in the left/right, up/down, and forward/backward directions.

Time

Time is a fourth dimension. In normal life, time is a tool we use to measure the procession of events of space. But time is something more. Yes, we use time as a "tool", but time is essential for our physical existence. Space and time when used to describe events can't be clearly separated. Therefore, space and time are woven together in a symbiotic manner. Having one without the other has no meaning in our physical world. To be redundant, without space, time would be useless to us and without time, space would be useless to us. This mutual dependence is known as the Spacetime Continuum. It means that any occurrence in our universe is an event of Space and Time. In Special Relativity, spacetime does not require the notion of a universal time component. The time component for events that are viewed by people in motion with respect to each other will be different. As you will see later, spacetime is the death of the concept of simultaneity.

Matter

Matter in the most fundamental definition is anything that takes up space. Any object you can see, touch, or move by applying a force is matter. Most people probably remember from school that matter is made up of millions of billions of tightly packed atoms. Water, for example, is the compound H2O, meaning two hydrogen atoms combined with one oxygen atom forms one molecule of water.

To fully understand matter let's look at the atom. It is now generally accepted that atoms are made up of three particles called neutrons, protons, and electrons. The neutrons and protons are found in the nucleus (center) of the atom and the electrons reside in a shell surrounding the nucleus. Neutrons are heavy particles, but they have no charge - they are neutral. Protons are also heavy particles and they have a positive charge. Electrons are light particles and they are negatively charged. There are many important features that arise from considering the number of these particles in each atom. For example, the number of protons an atom has will determine the atom's place on the periodic table, and it will determine how the atom behaves in the physical universe. (See the HSW article entitled "How Nuclear Radiation Works" for a further discussion of atoms and subatomic particles.)

Motion

Anything that is in the act of changing its location in space is said to be in motion. As you will see later, consideration of "motion" allows for or causes some very interesting concepts.

In the next section we'll look at mass and energy.

Mass has two definitions that are equally important. One is a general definition that most high school students are taught and the other is a more technical definition that is used in physics.

Generally, mass is defined as the measure of how much matter an object or body contains - the total number of sub-atomic particles (electrons, protons and neutrons) in the object. If you multiply your mass by the pull of earth's gravity, you get your weight. So if your body weight is fluctuating, by eating or exercising, it is actually your mass that is changing. It is important to understand that mass is independent of your position in space. Your body's mass on the moon is the same as its mass on the earth. The earth's gravitational pull, on the other hand, decreases as you move farther away from the earth. Therefore, you can lose weight by changing your elevation, but your mass remains the same. You can also lose weight by living on the moon, but again your mass is the same.

In physics, mass is defined as the amount of force required to cause a body to accelerate. Mass is very closely related to energy in physics. Mass is dependent on the body's motion relative to the motion of an observer. If the body in motion measured its mass, it is always the same. However, if an observer that is not in motion with the body measures the body's mass, the observer would see an increase in mass when the object speeds up. This is called relativistic mass. It should be noted that physics has actually stopped using this concept of mass and now deals mostly in terms of energy (see the section on the unification of mass and energy) . At this stage, this definition of mass may be a little cloudy, but it is important to know the concept. It should become clearer in the special relativity discussion. The important thing to understand here is that there is a relationship between mass and energy.

Energy

Energy is the measure of a system's ability to perform "work". It exists in many forms…potential, kinetic, etc. The law of conservation of energy tells us that energy can neither be created nor destroyed; it can only be converted from one form to another. These separate forms of energy are not conserved, but the total amount of energy is conserved. If you drop a baseball from your roof, the ball has kinetic energy the moment it starts to move. Just before you dropped the ball, it had only potential energy. As the ball moves, the potential energy is converted into kinetic energy. Likewise, when the ball hits the ground, some of its energy is converted to heat (sometimes called heat energy or heat kinetic energy). If you go through each phase of this scenario and totaled up the energy for the system, you will find that the amount of energy for the system is the same at all times.

In the next section we'll look at the properties of light.

Light is a form of energy, and exists in two conceptual frameworks: light exhibits properties that have characteristics of discrete particles (eg. energy is carried away in "chunks") and characteristics of waves (eg. diffraction). This split is known as duality. It is important to understand that this is not an "either/or" situation. Duality means that the characteristics of both waves and particles are present at the same time. The same beam of light will behave as a particle and/or as a wave depending on the experiment. Furthermore, the particle framework (chunks) can have interactions which can be described in terms of wave characteristics and the wave framework can have interactions that can be described in terms of particle characteristics. The particle form is known as a photon, and the waveform is known as electromagnetic radiation. First the photon…

A photon is the light we see when an atom emits energy. In the model of an atom, electrons orbit a nucleus made of protons and neutrons. There are separate electron levels for the electrons orbiting the nucleus. Picture a basketball with several sizes of hula-hoops around it. The basketball would be the nucleus and the hula-hoops would be the possible electron levels. These surrounding levels can be referred to as orbitals. Each of these orbitals can only accept a discrete amount of energy. If an atom absorbs some energy, an electron in an orbital close to the nucleus (a lower energy level) will jump to an orbital that is farther away from the nucleus (a higher energy level). The atom is now said to be excited. This excitement generally will not last very long, and the electron will fall back into the lower shell. A packet of energy, called a photon or quanta, will be released. This emitted energy is equal to the difference between the high and low energy levels, and may be seen as light depending on its wave frequency, discussed below.

The wave form of light is actually a form of energy that is created by an oscillating charge. This charge consists of an oscillating electric field and an oscillating magnetic field, hence the name electromagnetic radiation. We should note that the two fields are oscillating perpendicular to each other. Light is only one form of electromagnetic radiation. All forms are classified on the electromagnetic spectrum by the number of complete oscillations per second that the electric and magnetic fields undergo, called frequency. The frequency range for visible light is only a small portion of the spectrum with violet and red being the highest and lowest frequencies respectively. Since violet light has a higher frequency than red, we say that it has more energy. If you go all the way out on the electromagnetic spectrum, you will see that gamma rays are the most energetic. This should come as no surprise since it is commonly known that gamma rays have enough energy to penetrate many materials. These rays are very dangerous because of the damage they can do to you biologically (See the HSW article entitled "How Nuclear Radiation Works" for a further discussion of gamma radiation.). The amount of energy is dependent on the frequency of the radiation. Visible electromagnetic radiation is what we commonly refer to as light, which can also be broken down into separate frequencies with corresponding energy levels for each color.

As light travels its path, through space, it often encounters matter in one form or another. We should all be familiar with reflection since we see bright reflections when a light hits a smooth shiny surface like a mirror. This is an example of light interacting with matter in a certain way. When light travels from one medium to another, the light bends. This is called refraction. If the medium, in the path of the light, bends the light or blocks certain frequencies of it, we can see separate colors. A rainbow, for example, occurs when the sun's light becomes separated by moisture in the air. The moisture bends the light, thus separating the frequencies and allowing us to see the unique colors of the light spectrum. Prisms also provide this effect. When light hits a prism at certain angles, the light will refract (bend), causing it to be separated into its individual frequencies. This effect occurs because of the shape of the prism and the angle of the light.

If you look closely at what happens as the light wave enters the prism in the second diagram, you will notice that it bends down. This bending occurs because the light travels faster through the air than it does through the prism. When the lower portion of the wave enters the prism, it slows down. Since the upper portion of the wave (still in the air) is traveling faster than the lower portion, the wave bends. Similarly, as the wave exits the prism, the upper portion exits first and begins travelling faster than the lower portion that is still in the prism. This speed differential causes the wave to bend once again. Think of a skateboard rider going down the driveway. If the rider turns and goes into the grass, his body will lunge forward and actually fly off of the board if he is traveling fast enough originally. This is analogous to light bending as it goes through different mediums. The skateboard and the rider are moving at the same speed until the wheels hit the grass. Now suddenly, the skateboard is traveling slower than the rider is, so the rider begins to bend forward (the rider is trying to continue traveling at the same speed he was before the wheels hit the grass).

Now that we have a little understanding of the composition of light, we can begin to resolve the oft under explained concept of "the speed of light". Since light itself is just a form of electromagnetic radiation, the speed of light is just an easy way of talking about the speed of electromagnetic radiation in general. If you think about it, the speed of light is the "speed of information". We can not acknowledge that an event has occurred until the information about that event reaches us. The information is contained in the electromagnetic radiation from the event via a radio signal, a flash of light etc. Any event is just an occurrence of space and time, and any information that can be transmitted about an event is emitted outward as radiation of some sort. The information (electromagnetic radiation) from the event travels at 186,000 miles/second in a vacuum. If you picture a long train that begins to move forward from a stopped position, you do not expect the very last car to begin moving instantaneously. There is an amount of time that passes before the last car begins to get pulled. Thus, there is an expected delay for last car to "receive" the information that the first car is moving and pulling. This delay is analogous to the transfer of information in special relativity, but SR only imposes an upper limit on the speed of the information; the speed of light. You can make the train example as detailed as you like, but regardless, you will always find that there can be no reaction without a time delay of at least the speed of light between the action and reaction. In the special relativity section we will further discuss the importance of this speed.

You are now familiar with the major players in the universe: space, time, matter, motion, mass, gravity, energy and light. The neat thing about Special Relativity is that many of the simple properties discussed in section 1 behave in very unexpected ways in certain specific "relativistic" situations. The key to understanding special relativity is understanding the effects that relativity has on each property.

Frames of Reference

Einstein's special theory of relativity is based on the idea of reference frames. A reference frame is simply "where a person (or other observer) happens to be standing". You, at this moment, are probably sitting at your computer. That is your current reference frame. You feel like you are stationary, even though you know the earth is revolving on its axis and orbiting around the sun. Here is an important fact about reference frames: There is no such thing as an absolute frame of reference in our universe. By saying absolute, what is actually meant is that there is no place in the universe that is completely stationary. This statement says that since everything is moving, all motion is relative. Think about it - the earth itself is moving, so even though you are standing still, you are in motion. You are moving through both space and time at all times. Because there is no place or object in the universe that is stationary, there is no single place or object on which to base all other motion. Therefore, if John runs toward Hunter, it could be correctly viewed two ways. From Hunter's perspective, John is moving towards Hunter. From John's perspective, Hunter is moving towards John. Both John and Hunter have the right to observe the action from their respective frames of reference. All motion is relative to your frame of reference. Another example: If you throw a ball, the ball has the right to view itself as being at rest relative to you. The ball can view you as moving away from it, even though you view the ball as moving away from you. Keep in mind that even though you are not moving with respect to the earth's surface, you are moving with the earth.

We'll look at the first postulate of special relativity in the next section.

The first postulate of the theory of special relativity is not too hard to swallow: The laws of physics hold true for all frames of reference. This is the simplest of all relativistic concepts to grasp. The physical laws help us understand how and why our environment reacts the way it does. They also allow us to predict events and their outcomes. Consider a yardstick and a cement block. If you measure the length on the block, you will get the same result regardless of whether you are standing on the ground or riding a bus. Next, measure the time it takes a pendulum to make 10 full swings from a starting height of 12 inches above its resting point. Again, you will get the same results whether you are standing on the ground or riding a bus. Note that we are assuming that the bus is not accelerating, but traveling along at a constant velocity on a smooth road. Now if we take the same examples as above, but this time measure the block and time the pendulum swings as they ride past us on the bus, we will get different results than our previous results. The difference in the results of our experiments occurs because the laws of physics remain the same for all frames of reference. The discussion of the Second Postulate will explain this in more detail. It is important to note that just because the laws of physics are constant, it does not mean that we will get the same experimental results in differing frames. That depends on the nature of the experiment. For example, if we crash two cars into each other, we will find that the energy was conserved for the collision regardless of whether we were in one of the cars or standing on the sidewalk. Conservation of energy is a physical law and therefore, must be the same in all reference frames.

The Second Postulate of the Special Theory of Relativity

The second postulate of the special theory of relativity is quite interesting and unexpected because of what it says about frames of reference. The postulate is: The speed of light is measured as constant in all frames of reference. This can really be described as the first postulate in different clothes. If the laws of physics apply equally to all frames of reference, then light (electromagnetic radiation) must travel at the same speed regardless of the frame. This is required for the laws of electrodynamics to apply equally for all frames.

We'll take a closer look at the second postulate in the next section.

This postulate is very odd if you think about it for a moment. Here is one fact you can derive from the postulate: Regardless of whether you are flying in an airplane or sitting on the couch, the speed of light would measure the same to you in both situations. The reason that is unexpected is because most physical objects that we deal with in the world add their speeds together. Consider a convertible approaching you at a speed of 50 miles/hour. The passenger pulls out a slingshot and shoots a rock 20 miles/hour at you. If you measured the speed of the rock, you would expect it to be traveling at 70 miles/hour (the speed of the car plus the speed of the rock from the slingshot). That is, in fact, what happens. If the driver measured the speed of the rock, he would only measure 20 miles/hour, since he is already moving at 50 miles/hour with the car. Now if that same car is approaching you at 50 miles/hour and the driver turns on the headlights, something different happens? Since the speed of light is known to be 669,600,000 miles/hour, common sense tells us that the car's speed plus the headlight beam speed gives a total of 669,600,050 miles/hour (50 miles/hour + 669,600,000 miles/hour). The actual speed would measure 669,600,000 miles/hour, exactly the speed of light. To understand why this happens, we must look at our notion of speed.

Speed is the distance traveled in a given amount of time. For example, if you travel 60 miles in one hour, your speed is 60 miles per hour. We can easily change our speed by accelerating and decelerating. In order for the speed of light to be constant, even if the light is "launched" from a moving object, only two things can be happening. Either something about our notion of distance and/or something about our notion of time must be skewed. As it turns out, both are skewed. Remember, speed is distance divided by time.

We'll take a closer look at skewing in the next section.

In the headlight example, the distance that you are using in your measurement is not the same as the distance that the light is using. This is a very difficult concept to grasp, but it is true. When an object (with mass) is in motion, its measured length shrinks in the direction of its motion. If the object reaches the speed of light, its measured length shrinks to nothing. Only a person that is in a different frame of reference from the object would be able to detect the shrinking - as far as the object is concerned, in its frame of reference, its size remains the same. This phenomenon is referred to as "length contraction". It means, for example, that as your car approaches the speed of light, the length of the car measured by a stationary observer would be smaller than if the car was measured as it stood still. Look at Fig 2 and Fig 3 below.

In Fig 2 the car is stopped at the stop sign. In Fig 3 the same car is moving past you. You will readily notice that the moving car in the figure is shorter than the stopped car. Note that the car would only be shorter in the direction it is traveling, its height and width are not affected - only its length. Length contraction only affects the length in the direction you are traveling. Imagine that you are running super fast toward an open door. From your perspective, the distance from the front of the door opening to the back of the door opening would decrease. From the doors perspective the width of your body - the distance from your chest to your back - would decrease.

Scientists feel that they have actually proved this notion of length contraction. Therefore, in reality, all objects are perceived to shorten in the direction they are traveling, if they are viewed by someone who is not in motion with them. If you are in a moving car and measure the length of the armrest, you will never notice the change regardless of how fast you are going, because your tape measure would also be shortened from the motion.

In our lives we do not ever perceive length contraction because we move at speeds that are very small with respect to the speed of light. The change is too small for us to notice. Remember the speed of light is 669,600,000 miles/hour or 186,400 miles/sec, so it is easy to see why our everyday speeds are negligible.

We'll look at length contraction in the next section.

The Lorentz Transforms allow us to calculate the length contraction. How much contraction occurs is dependent on how fast an object is traveling with respect to the observer. Just to put some numbers to this, assume that a 12-inch football flies past you and it is moving at a rate of 60% the speed of light. You would measure the football to be 9.6 inches long. So at 60% the speed of light, you measure the football to be 80% of its original length (original 12 inch measurement was made at rest with respect to you). Keep in mind that all measurements are in the direction of the motion - The diameter of the ball is not changed by the ball's forward motion. Here are two points to keep in mind:

The Effect of Motion on Time

I mentioned that time also changes with different frames of reference (motion). This is known as "time dilation". Time actually slows with motion but it only becomes apparent at speeds close to the speed of light. Similar to length contraction, if the speed reaches that of light, time slows to a stop. Again, only an observer that is not in motion with the time that is being measured would notice. Like the tape measure in length contraction, a clock in motion would also be affected so it would never be able to detect that time was slowing down (remember the pendulum). Since our everyday motion does not approach anything remotely close to the speed of light, the dilation is completely unnoticed by us, but it is there.

We'll take a closer look at time dilation in the next section.

In order to attempt to prove this theory of time dilation, two very accurate atomic clocks were synchronized and one was taken on a high-speed trip on an airplane. When the plane returned, the clock that took the plane ride was slower by exactly the amount Einstein's equations predicted. Thus, a moving clock runs more slowly when viewed by a frame of reference that is not in motion with it. Keep in mind that when the clock returned, it had recorded less time than the ground clock. Once re-united with the ground clock, the slow clock will again record time at the same rate as the ground clock (obviously, it will remain behind by the amount of time it slowed on the trip unless re-synchronized). It is only when the clock is in motion with respect to the other clock that the time dilation occurs. Take a look at Fig 4 and Fig 5 below.

Let's assume that the object under the sun in Fig 4 is a light clock on wheels. A light clock measures time by sending a beam of light from the bottom plate to the top plate where it is then reflected back to the bottom plate. A light clock seems to be the best measure of time since its speed remains constant regardless of motion. So in Fig 4, we walk up to the light clock and find that it takes 1 sec for the light to travel from the bottom to the top and back to the bottom again. Now look at Fig 5. In this example, the light clock is rolling to the right, but we are standing still. If we could see the light beam as the clock rolled past us, we would see the beam travel at angles to the plates. If you are confused, look at Fig 4 and you'll see that both the sent beam and received beam occur under the sun, thus the clock is not moving. Now look at fig 5, the sent beam occurs under the sun, but the reflected beam returns when the clock is under the lightning bolt, thus the clock is rolling to the right. What is this telling us? We know that the clock standing still sends and receives at 1-second intervals. We also know that the speed of light is constant. Regardless of where we are, we would measure the light beam in fig 4 and fig 5 to be the exact same speed. But Fig 5 looks like the light traveled farther because the arrows are longer. And guess what, it did. It took the light longer to make one complete send and receive cycle, but the speed of the light was unchanged. Because the light traveled farther and the speed was unchanged, this could only mean that the time it took was longer. Remember speed is distance / time, so the only way for the speed to be unchanged when the distance increases is for the time to also increase.

We'll look at time intervals in the next section. 

Using the Lorentz Transform, let's put numbers to this example. Let's say the clock in Fig 5 is moving to the right at 90% of the speed of light. You, standing still, would measure the time of that clock as it rolled by to be 2.29-seconds. It is important to note that anyone in motion with the clock in Fig 5 would only measure 1-second, because it would be no different than him standing beside the clock in Fig 4. Hence, the rider aged by 1 second but you aged by 2.29 seconds. This is a very important concept. If we look closely at the clocks, we find that they do not really measure what we think they do. Clocks record the interval between two spatial events. This interval may differ depending on what coordinate system the clock is in (ie. what frame of reference). If the speed of light is held constant (has the same measured value regardless of frame of reference), time is no longer "just" a tool to measure the procession of space. It is a property that is required for the defining and existence of the event. Remember from earlier, any occurrence is an event of space and time (hence, the Space-Time Continuum).

[Note: If the reader decides to learn more about time dilation, it is absolutely imperative that strong emphasis be put on "proper time". This concept is not discussed in this article, but "proper time" is the foundation of the frame geometry of SR. This topic is clearly derived and discussed in the book Spacetime Physics by Taylor and Wheeler.]

The Unification of Energy and Mass

Undoubtedly the most famous equation ever written is E=mc². This equation says that energy is equal to the rest mass of the object times the speed of light squared (c is universally accepted as the speed of light). What is this equation actually telling us? Mathematically, since the speed of light is constant, an increase or decrease in the system's rest mass is proportional to an increase or decrease in the system's energy. If this relationship is then combined with the law of conservation of energy and the law of conservation of mass, an equivalence can be formed. This equivalence results in one law for the conservation of energy and mass. Let's now take a look at a couple examples of this relationship...

You should readily understand how a system with very little mass has the potential to release a phenomenal amount of energy (in E=mc², c² is an enormous number). In nuclear fission, an atom splits to form two more atoms. At the same time, a neutron is released. The sum of the new atoms' masses and the neutron's mass are less than the mass of the initial atom. Where did the missing mass go? It was released in the form of heat - kinetic energy. This energy is exactly what Einstein's E=mc² predicts. Another nuclear event that corresponds with Einstein's equation is fusion. Fusion occurs when lightweight atoms are subjected to extremely high temperatures. The temperatures allow the atoms to fuse together to form a heavier atom. Hydrogen fusing into helium is a typical example. What is critical is the fact that the mass of the new atom is less than the sum of the lighter atoms' masses. As with fission, the "missing" mass is released in the form of heat - kinetic energy.

One often-misinterpreted aspect of the energy-mass unification is that a system's mass increases as the system approaches the speed of light. This is not correct. Let's assume that a rocket ship is streaking through space. The following occurs:

In step 2, the system's resistance to acceleration is a measurement of the system's energy and momentum. Take notice that in the above 4 steps, there is no reference to mass. Nor does there need to be.

Next, we’ll look at why simultaneity between two events cannot happen in the world of special relativity.

There is no such thing as simultaneity between two events when viewed in different frames of reference. If you understand what we have talked about so far, this concept will be a breeze. First let's clarify what this concept is stating. If Meagan sees two events happen at the same time for her frame of reference, Garret, who is moving with respect to Meagan, will not see the events occur at the same time. Let's use another example. Imagine that Meagan is standing outside and notices that there are two identical cannons 100 yards apart and facing each other. All of the sudden, both cannons fire at the same time and the cannonballs smash into each other at exactly half their distance, 50 yards. This is no surprise since, the cannons are identical and they fire cannonballs at the same speed. Now, suppose that Garret was riding his skateboard super fast towards one of the cannons, and he was directly in the line of fire for both. Also suppose he was exactly half way between the two cannons when they fired. What would happen? The cannonball that Garret was moving towards would hit him first. It had less distance to travel since he was moving towards it.

Now, let's replace the cannons with light bulbs that turn on at the same time in Meagan's frame of reference. If Garret rides his skateboard in the same fashion as he did with the cannonballs, when he reaches the halfway mark, he sees the light bulb he is moving towards turn on first and then he sees the light bulb he is moving away from turn on last. See Fig 6 below for clarification.

In Fig 6, the bulb on the right turns on first. I have shown Garret to be moving in the same direction of the distance line between the bulbs, and he is looking towards the moon. As stated earlier, when the bulbs turn on in Meagan's frame of reference, Garret will see the bulb on the right turn on before the bulb on the left does. Since he is moving toward the bulb on the right, its light has a shorter distance to travel to reach him. Garret would argue with Meagan that the bulbs did not turn on at the same time, but in Meagan's perspective they did. Hopefully, you can see how different frames of reference will not allow events to be observed as simultaneous.

The Infamous Twin Paradox

Since SR dictates that two different observers each have equal right to view an event with respect to their frames of reference, we come to many not-so-apparent paradoxes. With a little patience, most of the paradoxes can be shown to have logical answers that agree with both the predicted SR outcome and the observed outcome. Let's look the most famous of these paradoxes - The Twin Paradox.

Suppose two twins, John and Hunter, share the same reference frame with each other on the earth. John is sitting in a spaceship and Hunter is standing on the ground. The twins each have identical watches that they now synchronize. After synchronizing, John blasts off and speeds away at 60% the speed of light. As John travels away, both twins have the right to view the other as experiencing the relativistic effects (length contraction and time dilation). For the sake of simplicity, we will assume that they have an accurate method with which to measure these effects. If John never returns, there will never be an answer to the question of who actually experienced the effects. But what happens if John does turn around and return to the earth? Both would agree that John aged more slowly than Hunter did, thus time for John was slower than it was for Hunter. To prove this, all they have to do is look at their watches. John's watch will show that it took less time for him to go and return than Hunter's watch shows. As Hunter stood there waiting, time passed faster for him than it did for John. Why is this the case if both were traveling at 60% the speed of light with respect to one another?

The first point to understand is that acceleration in SR is a little tricky (it's actually handled better in Einstein's Theory of General Relativity - GR). I don't mean to say that SR can't handle acceleration, because it can. In SR, you can describe the acceleration in terms of locally "co-moving" inertial frames. This allows SR to view all motion to be uniform, meaning constant velocity (non-accelerating). The second point is that SR is a "special" theory. By this, I mean that it is applicable in situations where there is no gravity, hence where space-time is flat. In GR, Einstein unifies acceleration and gravity so actually my previous statement is redundant. Anyway, the lack of gravity in SR is why it is called "Special Relativity". Now, back to the paradox… While both did view the other as shrinking and slowing down, the person that actually underwent the acceleration to reach the high speed is the one that aged less. If you dig deeper into the world of SR, you will realize that it's not really the acceleration that is important; it's the change of frame. Until John and Hunter returned to a frame of reference where their relative motion was zero (where they are standing beside each other) they would always disagree with what the other said he saw. As strange as this seems, there really isn't a conflict - both did observe that the other was experiencing the relativistic effects. One technique that is used to show the dynamics of the Twin Paradox is a concept is called the Relativistic Doppler Effect.

We'll look at the Doppler Effect in the next section.

The Doppler Effect basically says that there is an observed frequency shift in electromagnetic waves due to motion. The direction of the shift is dependent on whether the relative motion is traveling towards you or away from you (or vice versa). Also, the amplitude of the shift is dependent on the speed of the source (or the speed of the receiver). A good place to start in understanding the Doppler effect would be to first look at sound waves. There is a Doppler Shift associated with sound waves that you should recognize easily. When a sound source approaches you, the frequency of the sound increases and likewise, when the sound source moves away from you, the frequency of the sound decreases. Think about an approaching train blowing its whistle. As the train approaches, you hear the whistle tone as a high note. When the train passes you, you can hear the whistle tone change to a lower note. Another example occurs when cars race around a racetrack. You can hear a definite shift in the sound of the car as it passes where you are standing. One last example is the change in tone you hear when a police car passes you with its siren on. I'm sure that at some point in our lives, all of us have imitated the sound of a passing car or passing police car; we imitated the Doppler Shift. This Doppler shift also affects light (electromagnetic radiation) in the same manner with one critical exception; the shift will not allow you to determine if the light source is approaching you or if you are approaching the source and vice versa for moving away. This being said, let's look a fig 7 below.

In the top part of fig 7 you can see a stationary light source is emitting light in all directions. In the second part, you can see that source "S" is moving to the right and the light waves are shifted (they look as though they are being compressed in the front and dragged in the rear). If you approach the light source or the light source approaches you, the frequency of the light will appear to increase (notice that the waves in the front are closer together than in the rear). The opposite is true for a light source that is moving away from you or that you are moving away from. The importance of the frequency change is that if the frequency increases, then the time it takes for one complete cycle (oscillation) is less. Likewise, if the frequency decreases, the time it takes for one complete cycle is more.

Now let's apply this information to the Twin Paradox. Recall that John sped away from Hunter at 60% the speed of light. I picked this speed, because the corresponding relativistic Doppler shift ratio is "2 times" for an approaching source and "1/2" for a source that is moving away. This means that if the source is approaching you, the frequency will appear doubled (time is then halved) and if the source is moving away from you, the frequency will appear halved (time is then doubled). (similarly I could have used any speed for the paradox; for example, 80% the speed of light would have led to a Doppler shift of "3" and "1/3" for approaching and moving away respectively). Remember, the direction of the shift is dependent on the direction of the source, while the amplitude of the shift increases with the speed of the source.

We'll look at the Doppler shift in the next section.

Let's take another trip with the twins, but this time John will travel 12 hours away and 12 hours back, as measured by his clock. Every hour he will send a radio signal to Hunter telling him the hour. A radio signal is just another form of electromagnetic radiation; therefore, it also travels at the speed of light. What do we get as John travels away from Hunter? When John's clock reads "1 hour" he sends the first signal. Because he is moving away from Hunter at 60% of the speed of light, the relativistic Doppler Effect causes Hunter to observe John's transmission to be ½ the source value. From our discussion above, ½ the frequency means the time it takes is twice as long, therefore, Hunter receives the John's "1 hour" signal when his clock reads "2 hours". When John sends his "2 hour" signal, Hunter receives it at hour 4 for him. So you can see the relationship developing. For every 1-hour signal by John's watch, the elapsed time for Hunter is 2 hours. When John's clock reads "12 hours" he has sent 12 signals. Hunter, on the other hand, has received 12 signals, but they were all 2 hours apart … thus 24 hours have passed for Hunter. Now John turns around and comes back sending signals every hour in the same manner as before. Since he is approaching Hunter, the Doppler shift now causes Hunter to observe the frequency to be twice the source value. Twice the frequency is the same as ½ the time, so Hunter receives John's "1 hour" signals at 30min intervals. When the 12-hour return trip is over, John has sent 12 signals. Hunter has received 12 signals, but they were separated by 30 minutes, thus 6 hours have passed for Hunter. If we now total up the elapsed time for both twins, we see that 24 hours (12 + 12) have elapsed for John, but 30 hours (24 + 6) have elapsed for Hunter. Thus, Hunter is now older than his identical twin, John. If John had traveled farther and faster, the time dilation would have been even greater. Look at the twins again, but this time let John travel 84 hours out and 84 hours back (by his clock) at 80% the speed of light. The total trip for John will be 168 hours, and the total time elapsed for Hunter will be 280 hours; John was gone for 1 week by his clock, but Hunter waited for 1 week 4 days and 16 hours by his clock. Remember that Hunter will receive John's outgoing signals at half the frequency which means twice the time. Therefore, Hunter receives John's 84 hourly signals every 3 hours for a total of 252 hours (3 is the Relativistic Doppler shift for 80% the speed of light). Likewise, Hunter receives John's return trip 84 hourly signals every 20 minutes for a total of 28 hours (20 minutes is the 1/3 Relativistic Doppler shift for the return). Now you know the total round trip from Hunter's perspective, 252 + 28 = 280 hours or 1 week 4 days and 16 hours. John, on the other hand, traveled 84 hours out and 84 hours back for a total of 168 hours or 1 week.

We'll look closer at the twin paradox in the next section.

Now let's look at the twins again, but this time Hunter will send a signal every hour by his clock. What will John see? When Hunter sees the outgoing leg of John's trip end, his clock reads 15 hours and he has sent 15 signals. John, however, will say that he received 6 signals separated by 2-hours (relativistic Doppler shift) for a total of 12 hours. What happened to the other 9 signals? They are still in transit to John. Therefore, when John changes to his return leg, he will now encounter the missing 9 signals plus the 15 signals Hunter sent for the 15 hours his clock recorded for the return leg. So John receives 24 signals that are 30 minutes apart for a total of 12 hours. Like the previous example, these 24 signals have all been doppler shifted to a higher frequency because John is now approaching them. Now if we total the whole trip, Hunter sent one signal every hour for thirty hours, but John received 6 signals that were 2 hours apart and 24 signals that were 30 minutes apart. Hunter sent 30 signals in 30 hours; John received 30 signals in 24 hours. The result is the same as before, but the twins do not agree on when the first leg ended and the last leg began. So from this we can conclude that the change of frame for John (from outgoing to return) is what distinguishes him from Hunter. For Hunter, nothing changes at all. Anyway you look at it; he waits 30 hours without a change. John, however, does change. He changes from a frame in which he is moving away to a frame in which he is moving back. It is this change that breaks the symmetry between John and Hunter, thus removing the paradox as well.

Before going on to the next concept, I want to make sure that a couple things about SR and the speed of light are properly understood. First, SR predicts doom for anything with mass approaching the speed of light from a slower speed due to length contraction and time dilation, but it does allow for speeds greater than the speed of light. Consider the speed of light as a barrier. SR allows for existence on both sides of the barrier, but neither side can cross over to the other. As of yet, nothing has been discovered on the faster-than-light side, and all that we have are theories on particles (tachyons) that may have the ability to exist there. Maybe one day someone will discover their existence.

Secondly, velocities from a different frame of reference can not be summed. For example, if I run 5 miles/hour and at the same time, throw a rock 5 miles/hour, the only reason you (standing still) can say the rock is travelling 10 miles/hour is because the speed is so small with respect to the speed of light. We use the Lorentz Transformations to transform from one frame to another using the relative velocity of the frames. These transformations tell us mathematically that while at slow speeds the error in straight addition is much too small for us to detect, at very fast speeds, the error would become quite large. So classical mechanics, which teaches us to sum these velocities, is actually incorrect. We can do it, but it's a case of getting the right answer for the wrong reason.

We'll look at the twin paradox and simultaneity in the next section.

Simultaneity (or lack thereof) is a terrific tool for understanding many of the paradoxes associated with SR. And, if I am to be thorough, simultaneity must be considered for all SR events between separate frames of reference. Let's re-visit the twin paradox (John travels out 12 hours at 60% the speed of light and returns at the same speed). Basically, there are three frames of reference to consider. First, the twins are on the earth with no relative velocity between them. Second, John embarks on the outgoing leg of his trip. Thirdly, John (after instantaneously turning around) embarks on his return leg of his trip. I am using the same example as before, except I am using numbers from the Lorentz Transforms as opposed to the Relativistic Doppler Shift to explain the observed phenomena.

1st frame:

Hunter and John each agree on everything they observe. This should be easy to understand since there is no relative velocity between the two twins. They are in motion together.

2nd frame:

John travels out 12 hours by his clock. With the two postulates in mind, we realize that Hunter observes time dilation for John's outgoing trip. Thus, if John records 12 hours, Hunter will record 15 hours. Remember that at 60% the speed of light, the time dilation will be 80%. Therefore, if John records his time to be 12 hours, this is 80% of what Hunter records - 15 hours. But what does John observe for Hunter's time? He observes the time dilation as affecting Hunter; therefore, he measures his trip to be 12 hours, but he observes 9.6 hours (80% of his clock's time) for Hunter's time.

2nd frame totals:

Hunter measures his time to be 15 hours, but John's time to be 12 hours. John measures his time to be 12 hours, but Hunter's time to be 9.6 hours.

Obviously, the event, which is the end of the outgoing trip, is not simultaneous. John thinks Hunter's time is 9.6 hours but Hunter thinks his time is 15 hours. On top of that, they both think that John's time is 12 hours, which doesn't agree with either of the first two times.

We'll look at the results of this scenario in the next section. 

3rd frame:

From Hunter's perspective, nothing new has happened. He remained in his initial frame of reference and John returned at the same velocity he left with. Therefore, Hunter measured the return trip to take 15 hours for his frame (same as the outgoing trip) and observes the trip to take 12 hours for John. From John's perspective, he encountered a major change. He actually changed frames from one of traveling out to one of traveling back. Now, at the start of the return trip, when John looks at his clocks, he observes his clock to read 12 hours and Hunter's clock to read 20.4 hours. Think about this. John now shows that Hunter's clock has jumped ahead from 9.6 hours to 20.4 hours. How can this be???? When John changed from the 2nd frame to the 3rd frame, the established symmetry between Hunter and John was broken. Thus, each views their own time as having no change. And since John was the one that actually changed frames, he showed more elapsed time for Hunter. From here on out, it is business as usual. The return trip is clocked at 12 hours by John, but he observes 9.6 hours for Hunter. Again, let's clean this up…

3rd frame totals:

Hunter measures his time to be 15 hours, but he measures John's time to be 12 hours. John measures his time to be 12 hours, but he measures Hunter's time to be 9.6 hours. Remember, this 9.6 is only for the return trip after the frame change.

Trip totals:

Hunter measured his time to be 15 hours for the outgoing trip + 15 hours for the return trip…30 hours.

Hunter observed John's time to be 12 hours outgoing + 12 hours return …24 hours.

John measured his time to be 12 hours outgoing + 12 hours return…24 hours.

John observed Hunter's time to be 20.4 hours (after outgoing trip and frame change) + 9.6 hours for the return trip…20.4 + 9.6 = 30 hours.

Can you find any events in which both John and Hunter agree on the time for both themselves and the other? No, you can't. The lack of simultaneity is the key to the paradox. Both twins are measuring and observing. Unfortunately, they are not measuring and observing the same events. It is impossible for them to consider something like the end of the first leg as simultaneous when they each view it occurring at different times for Hunter. It's interesting to note that the results are the same as the Relativistic Doppler shift results. Is there a pattern here? SR allows for various methods to be employed to resolve the problems. For this case, use of space-time diagrams (there's those words again) would clearly show every point that we have talked about. I have merely used the Lorentz transforms in combination with the Relativistic Doppler effect.

We'll look at problems with the twin paradox in the next section.

Many people have trouble with the twin paradox because of the way in which the frame change is handled. In this case, the jump on John's clock for Hunter after the frame change (9.6 to 20.4 hours) is the problem. There really is no problem here. If you want to integrate the acceleration to use various inertial frames during the turn around, it can be done (with the same results). Another common approach is to imagine someone else in space that passes John just when he reaches the point of his turnaround. This person is heading towards Hunter at the same speed that John was travelling, so there is no need to consider John any further. The key fact is that if we then went back in the substitute's frame and looked at his clock for Hunter, it would show that some amount of time had already been recorded when the substitute began his trip towards Hunter. How far back should we go? Since John traveled out 12 hours on the outgoing trip, we should go back 12 hours in the substitute's frame. At this starting point for the substitute, his clock for Hunter would read 10.8 hours. This is extremely important. It clearly shows that both twins or the twin and the substitute observe the other as having slower times. The big shift occurs when the frame of reference is changed. This means that both observe the other to have a slower time during the actual outgoing and return trips, but there is a shift during the frame change that more than makes up for John's account of Hunter's slowly running clock. After the frame change, the damage has been done. John will still observe Hunter's clock to run slow, but it will never slow down enough to compensate for the 10.8 hours that were perceived during the frame change. Is this time jump a physical occurrence? No. The time jump occurs because when John changes frames, he is no longer using the same event as a reference. When John made his turnaround, the event in Hunter's frame that John thought was simultaneous with his turnaround changed. John's frame change caused this confusion because his new frame uses a different time for the event in Hunter's frame. More clearly, the turnaround event in Hunter's frame has a different time value for the outgoing leg and the return leg, as perceived by John. Keep in mind that in the above references to Hunter's frame, I'm really talking about what John thinks Hunter's frame time would be. This time difference is only apparent to John because it is his frame change that causes the discrepancy. In Hunter's frame, nothing changes for Hunter when John changes frames. Here again, by realizing that the two events are not simultaneous, the paradox is resolved. The point I am trying to emphasis is that there are a variety of ways to handle the paradox. All of the methods yield the same result, but if you actually consider the simultaneity of the situation, then the how's and why's become more clear.

We'll look at time travel in the next section.

Now that you have been introduced to the concepts of the theory, let's take a quick look at the relation between time travel and Special Relativity. If you remember the result from the twin paradox, you should agree that traveling into the future is possible, even at the speeds that our astronauts travel. Granted they would probably only be gaining a few nanoseconds, but when they return, the time on earth is ahead of their system time. Thus, they have returned to the future. As far as travelling back in time, Special Relativity is not as gracious as it is with moving forward. Let's take a look at this approach…

Many creative minds have wondered that since time slows down as you approach the speed of light, if you could find a way to travel faster than the speed of light, could you travel back in time? If I am to believe that special relativity is correct, then I am also to believe that the following events would occur. In order to travel faster than the speed of light, I assume that you would at some point have to travel at exactly the speed of light. For example, you can not travel 51 miles/hour without having traveled 50 miles/hour at some point, of course, this is providing that you were traveling 50 miles/hour or less to begin with. Now SR tells us that at the speed of light, time stops, your length contracts to nothing, and your resistance to acceleration becomes infinite requiring infinite energy (as observed by a frame of reference that is not in motion with the system). These conditions do not sound very conducive to life. Thus, I conclude that time travel into the past, using the concepts of SR, has some severe issues to overcome.

SR deals with contractions and dilations that are not in agreement with our commonsense views of the universe. In fact, they almost appear ludicrous. Yet, there have been several observations that agree with the predictions of SR. So, until the theory is proved wrong or a simpler theory produces the same results, SR will maintain its position as the best theory out there.

Here are five concepts you have discovered in this article:

As you pursue a better understanding of SR, Do Not fall prey to these errant statements:

The beauty in the theory of special relativity is that it gives us laws from which we can unite space and time and also energy and mass. Special relativity is definitely a thinking person's playground.

Special thanks to John M. Zavisa for contributing this article.