PEMDAS: Remembering Math's Order of Operations

PEMDAS is an acronym and mnemonic that represents a set of rules used to clarify the order in which operations should be performed to correctly evaluate mathematical expressions. PEMDAS stands for:

Sometimes, the mnemonic "BEDMAS" is used, where "B" stands for "brackets," and serves the same purpose as "parentheses." The mnemonics essentially convey the same order of operations to reach the correct answer, but they use slightly different terminology based on regional preferences. For example, BEDMAS is more commonly used in Canada, while PEMDAS is prevalent in the U.S.

(Note that multiplication and division are of equal precedence in the order of operations, so the flipped order in BEDMAS doesn't change anything.)

The order of operations — as Americans know it today — was probably formalized in either the late 18th century. By the 20th century, the tool gained wider acceptance, coinciding with the rise of the U.S. textbook industry.

In an email, math and science historian Judith Grabiner explains that concepts like the order of operations are best thought of as "conventions, like red-means-stop and green-means-go, not mathematical truths.

"But once the convention is established," she says, "the analogy to traffic lights holds: Everybody's got to do it the same way and the 'same way' has to be 100 percent unambiguous."

Math and ambiguity are uncomfortable bedfellows.

PEMDAS ensures consistency in the results of mathematical calculations. Basically, when different people evaluate the same expression, they use the same process and come to the same result. If you don't follow the correct order of operations, you will likely get the wrong answer.

Ignoring or changing this order can lead to different results, which can be especially problematic in fields like science, engineering and finance where precise calculations are crucial.

Suppose it's finals week, and you're expected to solve the following equation:

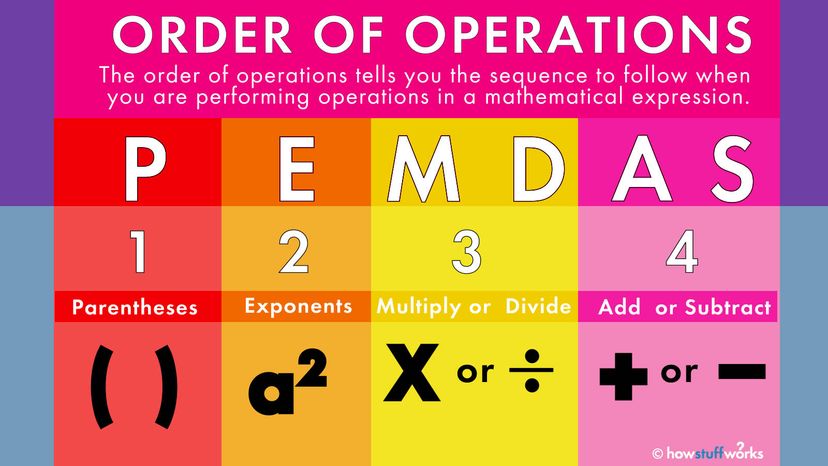
Don't panic. This is where a certain auntie comes in. For every word in the phrase, "Please excuse my dear Aunt Sally," there's a corresponding math term (which begins with the same letter) that tells us which procedure(s) to perform first.

Parentheses First

Before we solve the equation, PEMDAS dictates that we ask ourselves a simple question: "Are there any parentheses?" If the answer is "yes," then our first move should be to resolve whatever's inside them.

So in the above example, we see "2 x 3" enclosed in the parentheses. Therefore, we'll begin by multiplying 2 times 3, which gives us 6. Now the equation looks like this:

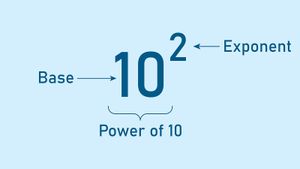
Cool beans. Time to bring on the exponents! In print, exponents take the form of a little number pressed against the upper righthand corner of a larger number. See the 5²? That itty-bitty "2" is an exponent.

Here, the tiny two tells us to multiply 5 by itself. And 5 x 5 equals 25, giving us this:

Now that we've taken care of the parentheses and exponent(s), let's proceed to those next two operations: multiplication and division.

Multiply and Divide

Note that we're not saying multiplication comes before division here. Not necessarily, at least.

Let's say you're looking at a different problem which — at this stage — contains both a multiplication sign and a division symbol. Your job would be to perform the two operations in order from left to right.

The concept is best explained by way of example. If the equation reads 8 ÷ 4 x 3, first you'd divide the 8 by the 4, giving you 2. Then — and only then — would you multiply that 2 by 3. We now return to our regularly scheduled math problem:

Whoever wrote the original equation kept things nice and simple; there's nary a division sign in sight and only one multiplication symbol. Thank you, merciful exam gods.

Without further ado, we're going to multiply the 6 by the 4, giving us 24.

Time to Add and Subtract

As with multiplication and division, addition and subtraction are part of the same step. Once again, we're performing these two operations in order, from left to right. So we're going to have to subtract that 24 from the 9.

Doing so will give us a negative number, specifically -15.

But the 25 is a positive number. So in its current form, the equation consists of a negative 15 plus a positive 25. And when you add those two together, you get a positive 10.

So there it is. The answer to our riddle.

Double Parentheses: Proceed With Care

Before we part ways, there are a few more things you should know. You may someday find yourself looking at a complex equation with lots of different operations sandwiched between two parentheses. Maybe something like this:

Don't sweat it. If you're trying to solve math problems with multiple operations, following the PEMDAS sequence ensures consistent and accurate results. All you've got to do is work through the PEMDAS process inside those parentheses before you move on to the rest of the problem.

Here, you'd take care of the exponent first (i.e., the 2³), then handle the subtraction in that set of parenthesis before moving on to the multiplication in the next level of parenthesis. Easy-peasy. (In case you're interested, the answer to the equation is 2 1/3, or 2.33 if you prefer decimals.)

Here are some other PEMDAS-eque conventions and methods related to arithmetic expressions:

This article was updated in conjunction with AI technology, then fact-checked and edited by a HowStuffWorks editor.