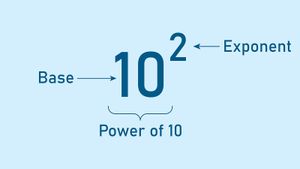
Key Takeaways
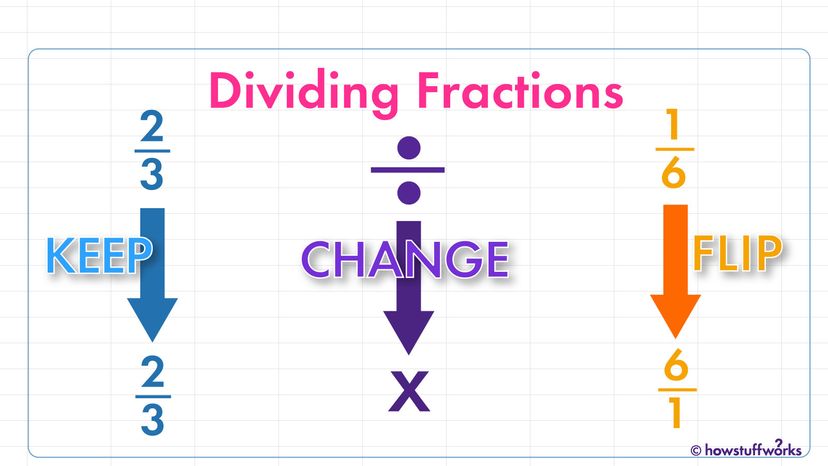
- To divide fractions, start by keeping the numerators and denominators of both fractions as they are.
- Change the division sign to a multiplication sign and find the reciprocal of the second fraction (the divisor) by flipping its numerator and denominator.
- After multiplying, simplify the resulting fraction if necessary to get your final answer.
The words "dividing fractions" can cause anxiety in almost anyone — you have to flip fractions and know words like divisor and dividend and reciprocal. The steps involved in dividing fractions may seem hard to remember, but they're easy with a little practice. Because math is all about remembering rules and terms, and if you can do that, dividing fractions is a breeze.
Division is the inverse of multiplication, so one thing you have to remember when dividing fractions is the answer is always going to be larger than either of the components of the problem. You're basically trying to figure out how many of the divisor (the second number in the problem) can be found in the dividend (the first number). If you know how to multiply fractions, you'll have no trouble learning how to divide fractions.
Advertisement
Advertisement