Key Takeaways
- The Pythagorean theorem is crucial in various fields, including construction, manufacturing and navigation, enabling precise measurements and the creation of right angles for large structures.
- It underpins our entire system of measurement, allowing for accurate navigation by pilots and ships, and making GPS measurements possible through the calculation of distances and angles.
- Beyond navigation, the theorem is essential in geometry, physics, geology, engineering and even practical applications by carpenters and machinists.
The Pythagorean theorem is an ancient mathematical theorem which is one of the most fundamental and important concepts in two-dimensional Euclidean geometry going back thousands of years. It can help students find the sides of a right triangle on a piece of paper, but it also has greater implications in the fields of engineering, physics and architecture.
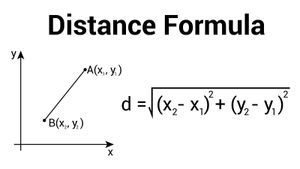
Since triangles always follow concrete rules we can use concepts like the Pythagorean theorem formula — and later, trigonometry — to find all the parameters of a triangle (angle values, lengths) if at least one of them is known.
Advertisement
The Pythagorean theorem is the simplest of these concepts and lets us easily solve the length of a third side of a right triangle if two sides are currently known.
Advertisement