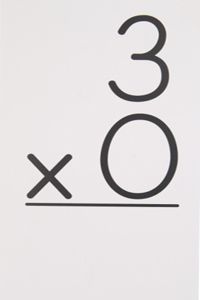How Zero Works

That zero manages to be both a non-negative and non-positive integer yet is neither negative nor positive is just one of the unique properties of the number. In fact, there is a group of these strange characteristics called the properties of zero.

The addition property of zero says that if you add or subtract zero from any other number, the answer will always result in the other number. 5+0=5 and 9,000,017-0=9,000,017, for example. It reflects the concept of zero as representing nothing -- so nothing added to something leaves that something unchanged -- zero is the only number that doesn't alter other numbers through addition or subtraction.

The additive inverse property of zero reflects its position as the fulcrum between the negative and positive integers. Any two numbers whose sum is zero are additive inverses of one another. For example, if you add -5 to 5, you arrive at zero. So -5 and 5 are additive inverses of one another.

The multiplication property states what every third-grader knows: Multiplying any number by zero results in a total of zero. It's obvious once ingrained but perhaps the reason is overlooked. Multiplication is, in one effect, a shortcut for addition. 3x2 is the same as 2+2+2, so the idea that a number can be added zero times or that zero can be added to itself any number of times is mathematically senseless [source: Carasco].

The concept of dividing by zero is even more senseless, so much so there is no property for it; the concept simply doesn't exist since it can't be carried out. Even mathematicians often struggle to explain why dividing by zero doesn't work. The reason is essentially related to the multiplication property. When dividing a number by another number, for example 6/2, the result (in this case, 3) can be meaningfully plugged into a formula where the answer multiplied by the divisor equals the dividend. In other words, 6/2=3 and 3x2=6. This doesn't work with zero when we replace 2 with it as the divisor; 3x0=0, not 6 [source: Utah Math]. The concept of dividing by zero is fraught with illogical consequences, so much so that its mythical destructive power has become a joke on the Internet.

There is also the property of the zero exponent; because of the existence of negative exponents, numbers to the negative power, numbers to the zero power always equal one. Although this works mathematically, it too presents logical problems. Chiefly, zero to the zero power still equals one, although zero added or subtracted to or multiplied by itself should equal zero [source: Stapel].

Behold, the power of zero.

Related Articles

Sources