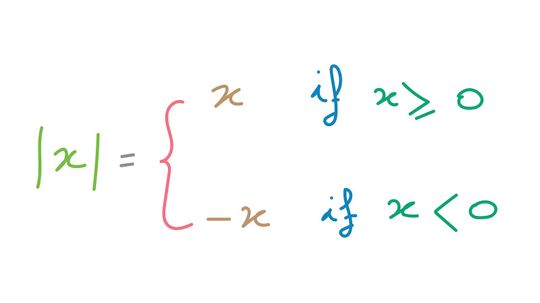Physical Science
Physical science is the study of the physical world around you. Learn about everything from electricity to magnetism in this section.
Learn More
From lifesaving medical components to everyday essentials, the most expensive liquid on Earth isn’t always what you'd expect. These liquids are priced by rarity, production difficulty, and real-world value — and some cost far more per liter than gold.
From cocktails to cooking, alcohol is everywhere. But not all alcohol is created equal. The types of alcohol range from drinkable spirits to industrial solvents, each with different uses, chemical properties and risks.
Ever wondered why some dead bodies last for thousands of years while others break down into dust? That's where the science of mummification comes in. Whether it's through ancient rituals, boggy swamps or some truly extreme self-imposed methods, mummified human remains give us an incredible look into history, biology and even chemistry.
Advertisement
"Why are there so many songs about rainbows?" sings Kermit the Frog in a song (about rainbows) called "Rainbow Connection" from 1979's "The Muppet Movie." And it's not just songs that feature rainbows. Children's memorabilia (from "My Little Pony" to Lisa Frank to anything with unicorns) and sweet treats ( from Lucky Charms to sherbert) are all filled with these dazzling rainbow colors.
By Ada Tseng
Ever wondered what the most abundant element on Earth is? Or how the elements that make up our planet compare to those floating out in the vast universe?
By Mack Hayden
Two congruent angles are simply pairs of angles with equal measures. You can find congruent angles examples in hundreds of everyday objects.
By Mitch Ryan
Adjacent angles are one of the earliest and most important concepts to learn in basic geometry, as they have applications in further subjects like trigonometry, physics, and engineering. Effective students of geometry should be able to identify adjacent angles on sight and calculate them to a great degree of accuracy without a protractor.
By Talon Homer
Advertisement
When pursuing an education in mathematics and algebra, one of the earliest and most important concepts to understand is the associative property, also known as the associative law.
By Talon Homer
In chemistry, there are a variety of methods that scientists use to identify a chemical compound, including a molecular formula, molar mass and molecular diagram. The simplest formula of these is known as the empirical formula.
By Talon Homer
In the subjects of geometry and trigonometry, a linear pair of angles is any two adjacent angles formed together to add up to 180°, or π (pi) radians.
By Talon Homer
Precious metals are not just shiny and attractive; these elements are also incredibly valuable, often used in a wide range of industries from jewelry to high-tech applications.
By Mack Hayden
Advertisement
With its three sides and three angles, the triangle is one of the most basic shapes in geometry. This means calculating the area of a triangle is a fundamental skill in geometry, with multiple formulas available depending on the type of triangle and the given data.
By Marie Look
In your study of math, you will likely come across the concept of factors at a certain point. While this can be daunting if you've never encountered it before, it won't take long before you're able to determine all the factors of a particular number.
By Zach Taras
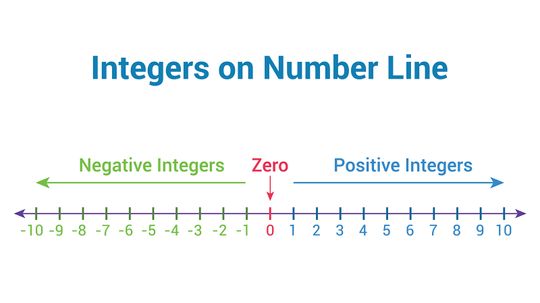
Integers are natural numbers (or whole numbers) that stem from the Latin word meaning "intact." In other words, any two integers will be rational numbers. A rational number is a value without fractional part or decimal remainders.
By Mitch Ryan
A rectangular prism is a three-dimensional cuboid figure. In the same way that a triangular prism brings three connected lines to life in the real world, a rectangular prism takes the length and width of two-dimensional rectangles to the next level by adding height into the equation.
By Mitch Ryan
Advertisement
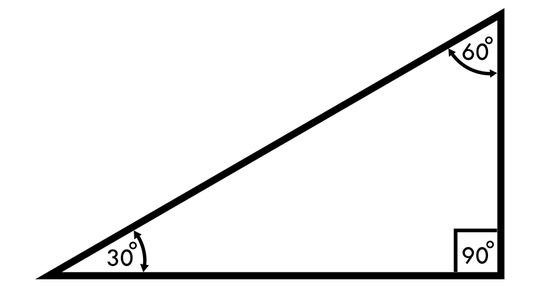
Anyone who's ever faced mind-numbing trigonometry problems and practice questions will be familiar with the Pythagorean Theorem and its square root principles in the 30-60-90 triangle. This special triangle has several short-cut rules to help new mathematicians find interior angles and linear lengths quickly.
By Mitch Ryan
In algebra and calculus, a polynomial function is used to chart out graphs and waves with much more complexity than a simple linear factor. Polynomial division is sometimes required to factor them, and cut them up into chunks that we humans can better understand.
By Talon Homer
Arcs are an important aspect of geometry, physics, trigonometry and design work. However, curved lines are much more difficult to measure than straight lines, which is why it's important to familiarize yourself with the arc length formula.
By Talon Homer
The interquartile range (IQR) is a statistical measure of the middle values of a sample data set that is separated into four equal parts. This middle-value grouping can provide a median range between the upper half and lower half of the data you've collected, allowing you to ignore extreme values.
By Mitch Ryan
Advertisement
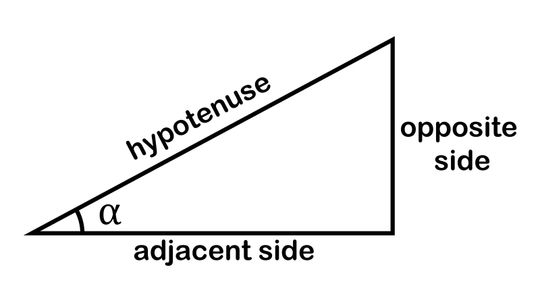
The mnemonic device SOHCAHTOA helps budding mathematicians remember the trigonometric functions sine (sin), cosine (cos) and tangent (tan), which they need to solve for triangles' missing sides and angles.
By Marie Look
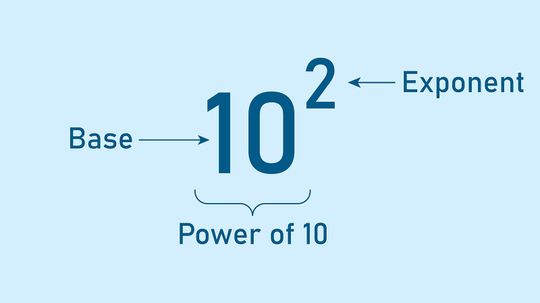
When you're dealing with exponents, numbers can get very big (or very small) very quickly. Therefore, it's helpful to have some short cuts.
By Zach Taras
Absolute value is a mathematical concept often used in conjunction with a number line or graph to represent the relative value from zero (modulus). To illustrate this idea in a different way, the absolute value of a number can be closely related to distance in the physical world.
By Mitch Ryan
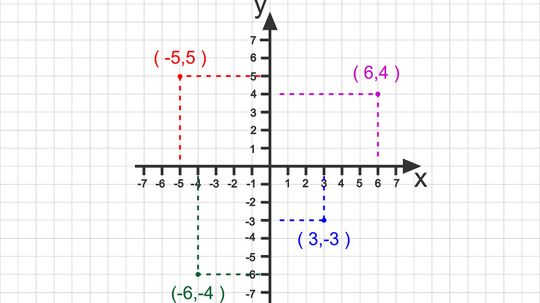
A coordinate plane is a mathematical, two-dimensional plane formed by two number lines. A horizontal number line and a vertical number line intersect to form two axes (plural for axis), and a grid system comprised of four quadrants.
By Mitch Ryan
Advertisement
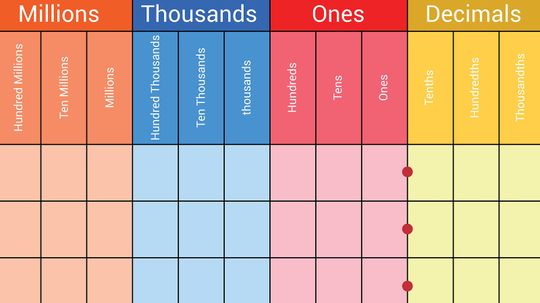
The concept of place values is fundamental to mathematics and the number system, particularly when you're dealing with whole numbers and decimal numbers. A place value chart is an essential tool that can help students visualize the position of each digit in a number and understand its actual value within the context of the number.
By Marie Look
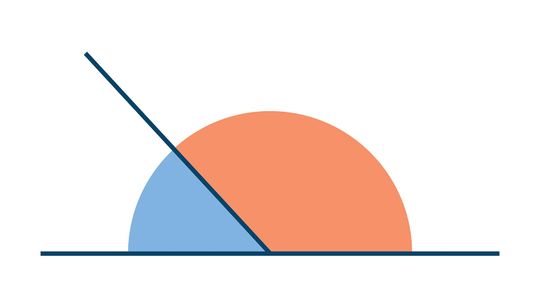
You've probably seen supplementary angles examples in your everyday life without knowing it. Whether you pass a leaning sign on a flat highway or walk by a shed with a lean-to roof - whenever two angles combine to form a straight, linear pair, there they are.
By Mitch Ryan